30-second summary
Electric Flux
The electric flux through a planar area is defined as the electric field times the component of the area perpendicular to the field.
If the area is not planar, then the evaluation of the flux generally requires an area integral since the angle will be continually changing.
Electric flux depends on the strength of electric field, E, on the surface area, and on the relative orientation of the field and surface. For a uniform electric field E passing through an area A, the electric flux E is defined as:
Φ = E x A

About Electric Flux
Gauss’s law involves the concept of electric flux, which refers to the electric field passing through a given area. In words:
Gauss’s law states that the net electric flux through any hypothetical closed surface is equal to 1/ε0 times the net electric charge within that closed surface.
ΦE = Q/ε0
In pictorial form, this electric field is shown as a dot, the charge, radiating “lines of flux”. These are called Gauss lines. Note that field lines are a graphic illustration of field strength and direction and have no physical meaning. The density of these lines corresponds to the electric field strength, which could also be called the electric flux density: the number of “lines” per unit area. Electric flux is proportional to the total number of electric field lines going through a surface.
Electric flux depends on the strength of electric field, E, on the surface area, and on the relative orientation of the field and surface. For a uniform electric field E passing through an area A, the electric flux E is defined as:
Φ = E x A
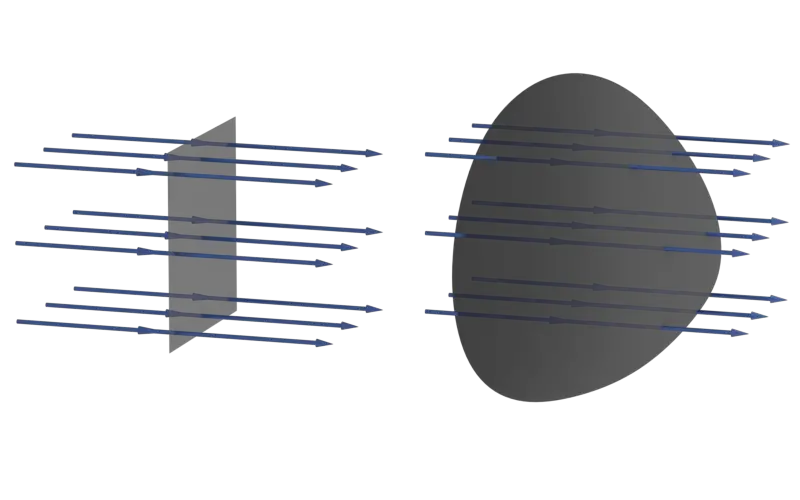
This is for the area perpendicular to vector E. We generalize our definition of electric flux for a uniform electric field to:
Φ = E x A x cosφ (electric flux for uniform E, flat surface)
What happens if the electric field isn’t uniform but varies from point to point over the area ? Or what if is part of a curved surface? For a non-uniform electric field, the electric flux dΦE through a small surface area dA is given by:
dΦE = E x dA
We calculate the electric flux through each element and integrate the results to obtain the total flux. The electric flux ΦE is then defined as a surface integral of the electric field:

Frequently asked questions
Gauss’s law is useful for determining electric fields when the charge distribution is highly symmetric. In choosing the surface, always take advantage of the symmetry of the charge distribution so that E can be removed from the integral.
Like Ampere’s law, which is analogous to magnetism, Gauss’ law is one of four Maxwell’s equations (the first) and thus fundamental to classical electrodynamics.
The coulomb (symbol: C) is the International System of Units (SI) unit of electric charge. The coulomb was defined as the quantity of electricity transported in one second by a current of one ampere: 1 C = 1 A × 1 s

