Equipotential surfaces are 3D spaces where every point has the same potential energy, either electric or gravitational, and are always perpendicular to field lines.
Understanding Equipotential Surfaces
Equipotential surfaces are essential concepts in physics, particularly in the study of electric and gravitational fields. In this article, we will explore the definition, properties, and examples of equipotential surfaces to provide a comprehensive understanding of the concept.
Definition of Equipotential Surfaces
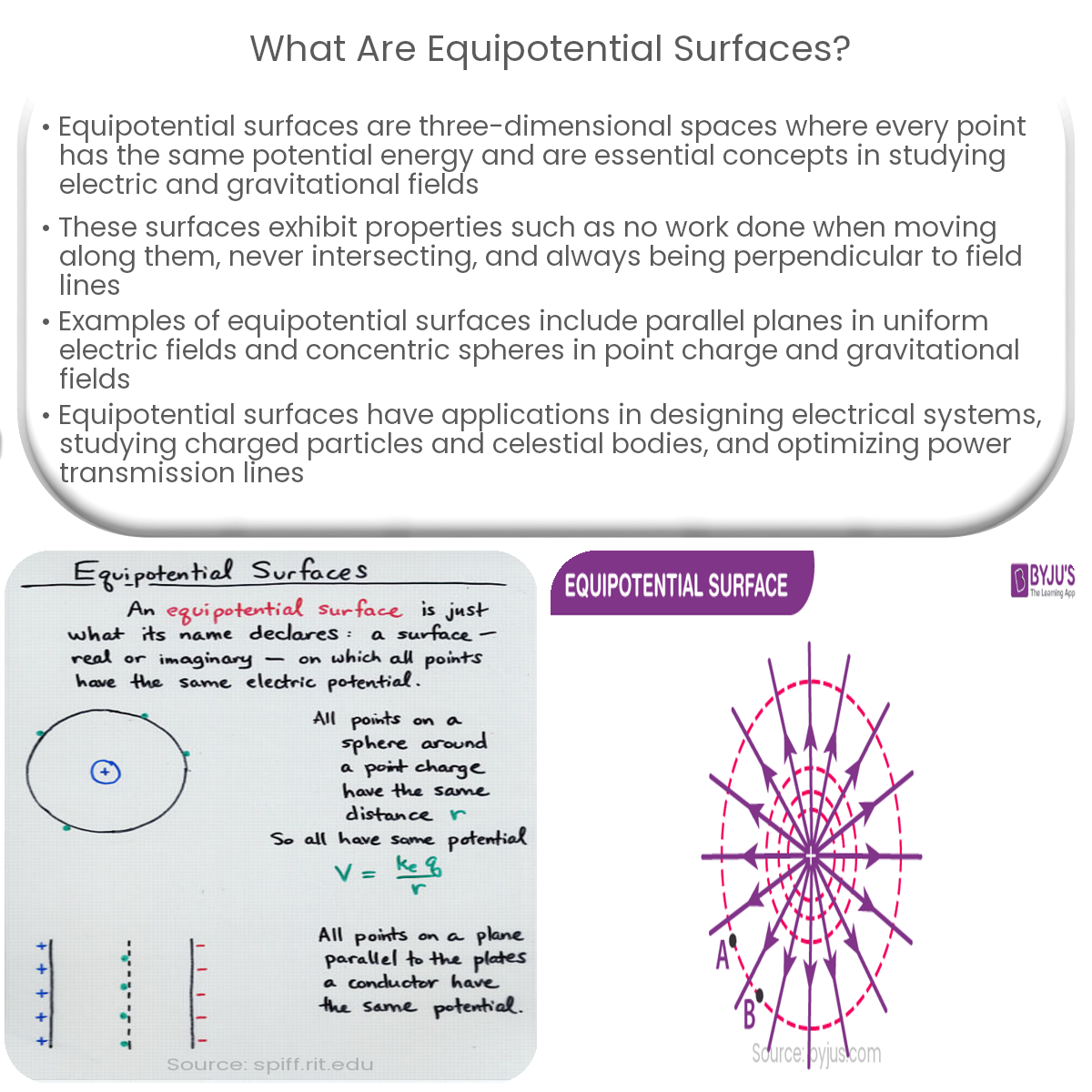
An equipotential surface is a three-dimensional space where every point on the surface has the same potential energy. In the context of electric fields, this refers to electric potential, while in gravitational fields, it refers to gravitational potential. Equipotential surfaces are always perpendicular to the field lines, which represent the direction of the force acting on a charged particle or mass.
Properties of Equipotential Surfaces
- No work is done when moving a charge or mass along an equipotential surface, as the potential energy remains constant.
- Equipotential surfaces never intersect, as each surface represents a unique potential energy value.
- Field lines are always perpendicular to equipotential surfaces. This property is crucial in understanding the relationship between the direction of force and potential energy.
- The spacing between equipotential surfaces indicates the strength of the field. Closely spaced surfaces represent a strong field, while widely spaced surfaces indicate a weak field.
Examples of Equipotential Surfaces
- Uniform Electric Field: In a uniform electric field, equipotential surfaces are evenly spaced parallel planes. The potential difference between any two adjacent planes remains constant.
- Point Charge: For a point charge, the equipotential surfaces are concentric spheres centered around the charge. The electric potential decreases as the distance from the charge increases, following an inverse-square law.
- Gravitational Field: In the case of a gravitational field surrounding a celestial body, the equipotential surfaces are also concentric spheres. The gravitational potential decreases as the distance from the mass increases, similarly obeying an inverse-square law.
Applications of Equipotential Surfaces
Equipotential surfaces have various applications in science and engineering, such as:
- Designing electrical systems to minimize energy loss.
- Understanding the behavior of charged particles in electric and magnetic fields.
- Studying the motion of celestial bodies in a gravitational field.
- Optimizing the layout of electric power transmission lines to maximize efficiency and safety.
In conclusion, equipotential surfaces play a vital role in comprehending the behavior of electric and gravitational fields. Understanding these surfaces allows us to predict the motion of charges and masses, leading to the development of efficient systems and improved understanding of the natural world.