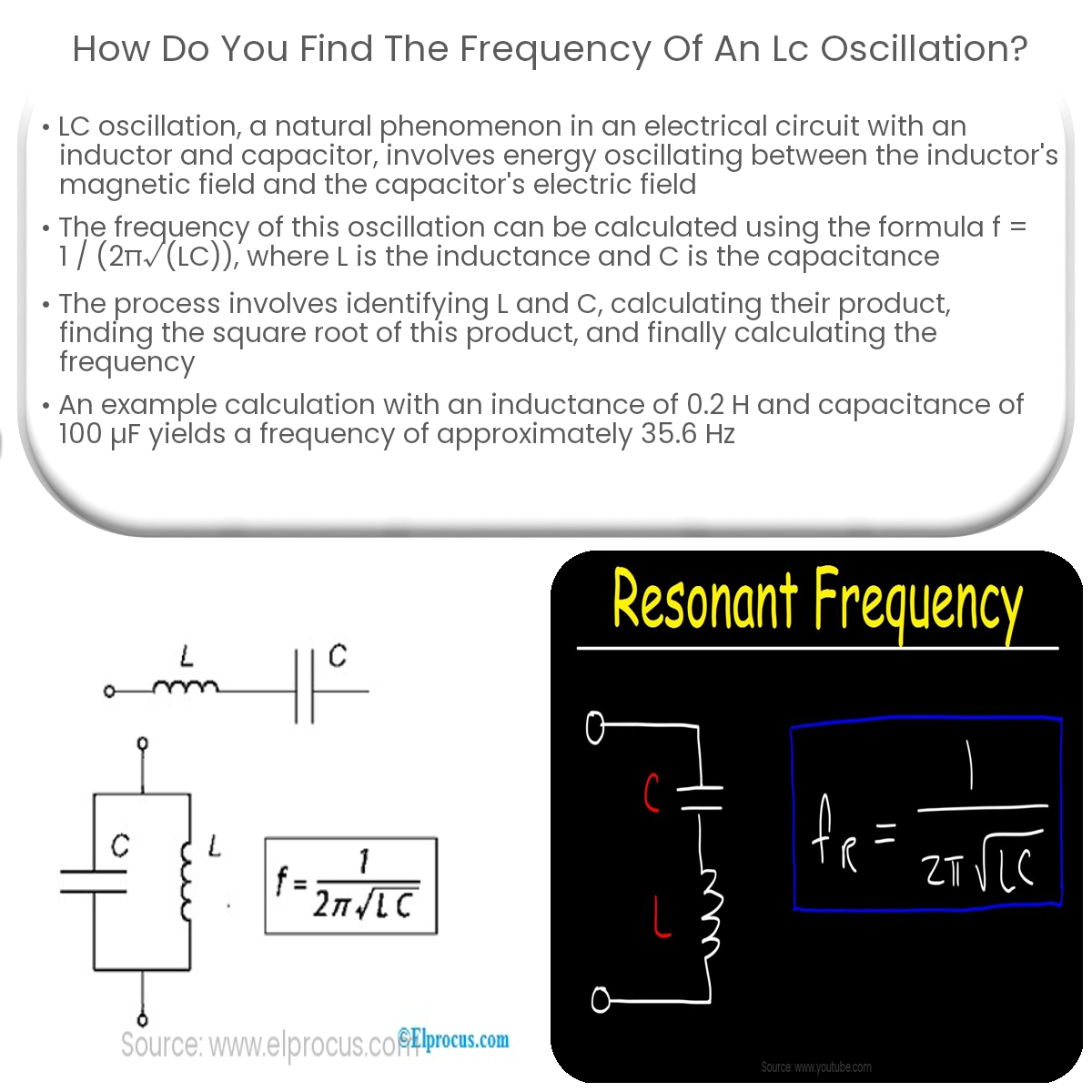
To find the frequency of an LC oscillation, use the formula f = 1 / (2π√(LC)), where L is inductance and C is capacitance. Calculate accordingly.
Finding the Frequency of an LC Oscillation
An LC oscillation is a natural phenomenon that occurs in an electrical circuit with an inductor (L) and capacitor (C) connected together. The energy oscillates between the inductor’s magnetic field and the capacitor’s electric field, resulting in oscillatory behavior. To analyze and design such circuits, it’s essential to know the frequency of the oscillation. In this article, we will discuss how to find the frequency of an LC oscillation.
LC Oscillation Frequency Formula
The frequency of an LC oscillation can be found using the following formula:
f = 1 / (2π√(LC))
where f is the frequency of the oscillation, L is the inductance of the inductor in henries (H), and C is the capacitance of the capacitor in farads (F).
Steps to Calculate the LC Oscillation Frequency
- Identify the inductance (L) and capacitance (C) values: To calculate the frequency, first determine the values of the inductor and capacitor in the circuit.
- Calculate the product of L and C: Multiply the inductor’s inductance (L) by the capacitor’s capacitance (C). This product represents the time constant of the LC circuit, which affects the frequency of oscillation.
- Find the square root of the product: Take the square root of the product obtained in step 2. This value is the reciprocal of the angular frequency (ω).
- Calculate the frequency (f): Finally, divide 1 by the product of 2π and the square root of the product of L and C. This will give you the frequency of the LC oscillation in hertz (Hz).
Example
Let’s consider an example to demonstrate the calculation of the LC oscillation frequency. Suppose we have an inductor with an inductance of 0.2 H and a capacitor with a capacitance of 100 µF (1 µF = 10-6 F). Following the steps mentioned above:
- L = 0.2 H, C = 100 µF = 100 × 10-6 F
- LC = 0.2 × 100 × 10-6 = 2 × 10-5
- √(LC) = √(2 × 10-5) ≈ 4.47 × 10-3
- f = 1 / (2π × 4.47 × 10-3) ≈ 35.6 Hz
Thus, the frequency of the LC oscillation in this example is approximately 35.6 Hz.