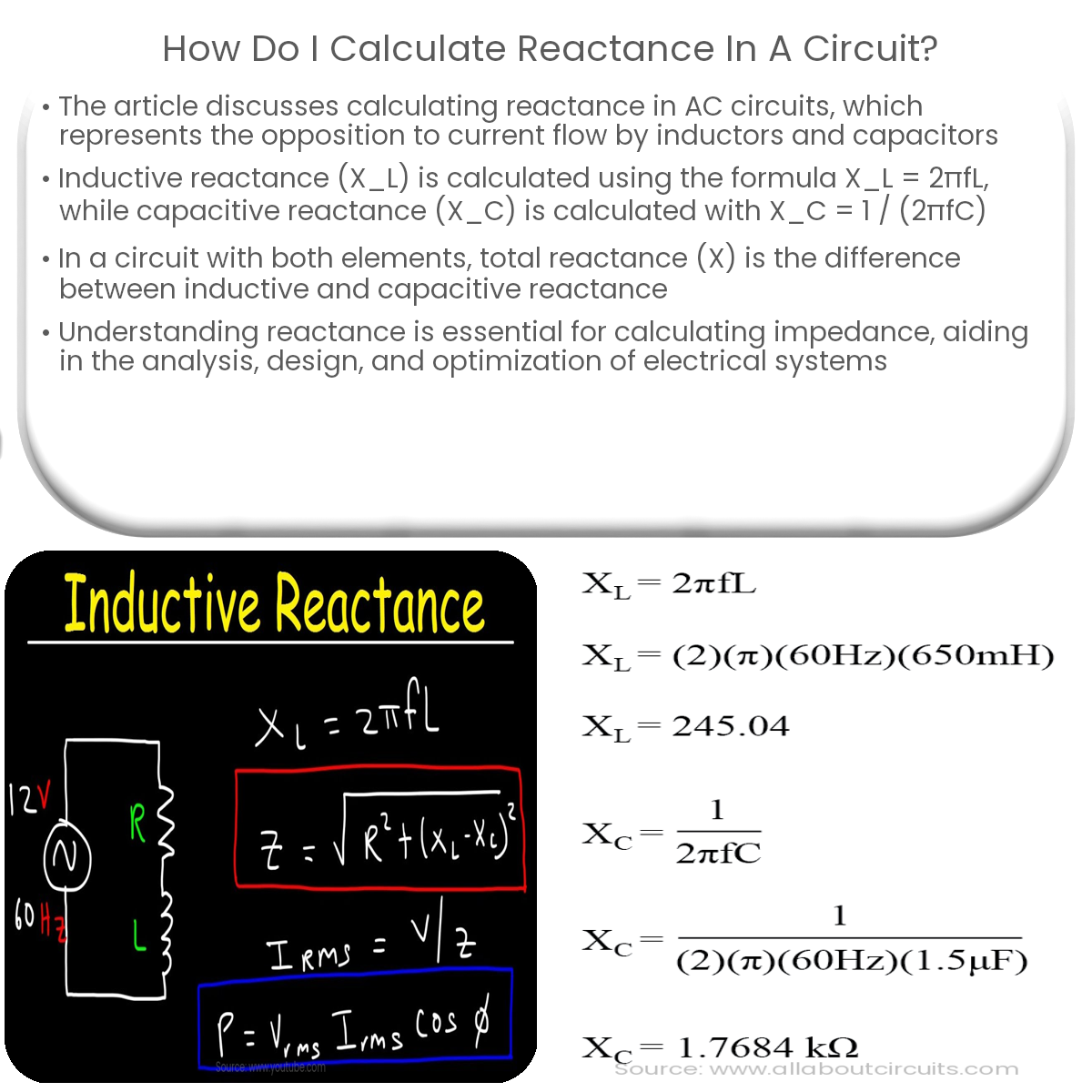
Calculate inductive reactance using X_L = 2πfL and capacitive reactance using X_C = 1 / (2πfC). Total reactance is the difference: X = X_L – X_C.
How to Calculate Reactance in a Circuit
Reactance is an essential concept in electrical engineering when working with alternating current (AC) circuits. It represents the opposition to current flow posed by inductors and capacitors. This article will discuss how to calculate inductive and capacitive reactance in a circuit.
Inductive Reactance
Inductive reactance (XL) is the opposition to AC current flow caused by an inductor. It depends on the frequency (f) of the AC signal and the inductance (L) of the inductor. To calculate inductive reactance, use the following formula:
XL = 2πfL
Where:
- XL is the inductive reactance
- f is the frequency in Hertz (Hz)
- L is the inductance in Henries (H)
Capacitive Reactance
Capacitive reactance (XC) is the opposition to AC current flow caused by a capacitor. It depends on the frequency (f) of the AC signal and the capacitance (C) of the capacitor. To calculate capacitive reactance, use the following formula:
XC = 1 / (2πfC)
Where:
- XC is the capacitive reactance
- f is the frequency in Hertz (Hz)
- C is the capacitance in Farads (F)
Calculating Total Reactance
In a circuit containing both inductive and capacitive elements, the total reactance (X) is the difference between inductive and capacitive reactance. Use the following formula:
X = XL – XC
Note that if the inductive reactance is greater than the capacitive reactance, the total reactance will be positive, indicating that the circuit is inductive. If the capacitive reactance is greater, the total reactance will be negative, indicating a capacitive circuit.
Using Reactance in Circuit Analysis
Calculating reactance helps in analyzing AC circuits, particularly when determining impedance (Z), which is the total opposition to current flow. Impedance consists of resistance (R) and reactance (X), and can be represented as a complex number:
Z = R + jX
Where j is the imaginary unit.
Knowing the impedance, you can calculate voltage, current, and power in AC circuits, enabling you to design and optimize various electrical systems.
Conclusion
Calculating reactance in a circuit is crucial for understanding the behavior of inductors and capacitors in AC circuits. With this knowledge, you can analyze, design, and optimize electrical systems involving these components.