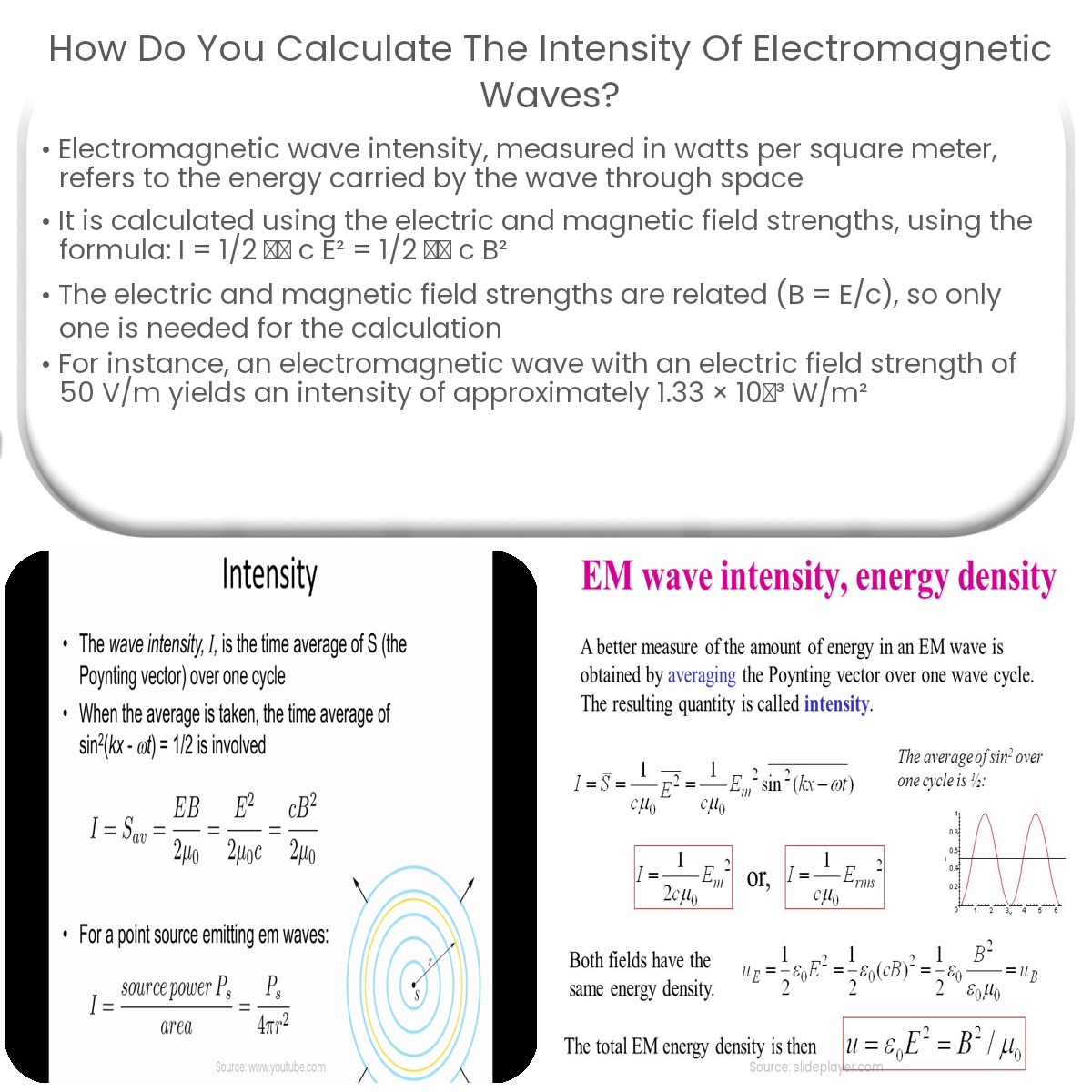
To calculate the intensity of electromagnetic waves, use the formula I = 1/2 μ₀ c E² or I = 1/2 ε₀ c B², with E or B field strength given.
Calculating the Intensity of Electromagnetic Waves
Electromagnetic waves are a fundamental aspect of our daily lives, from the visible light we see to the radio waves used in communication. Understanding the intensity of these waves is crucial to grasping their behavior and potential applications. In this article, we will discuss the formula used to calculate the intensity of electromagnetic waves and break down the key components involved in the process.
Intensity Definition
Intensity is defined as the amount of energy transmitted per unit area, per unit time. In the context of electromagnetic waves, intensity refers to the energy carried by the wave as it propagates through space. The unit of intensity is watts per square meter (W/m2).
Calculating Electromagnetic Wave Intensity
To calculate the intensity of an electromagnetic wave, we need to consider two primary factors: the electric field strength (E) and the magnetic field strength (B). The formula for intensity (I) is given by:
I = 1/2 μ0 c E2 = 1/2 ε0 c B2
Where:
- I is the intensity of the electromagnetic wave in watts per square meter (W/m2)
- μ0 is the permeability of free space, approximately equal to 4π × 10-7 N/A2
- ε0 is the permittivity of free space, approximately equal to 8.85 × 10-12 C2/N·m2
- c is the speed of light in a vacuum, approximately equal to 3 × 108 m/s
- E is the electric field strength in volts per meter (V/m)
- B is the magnetic field strength in tesla (T)
It is important to note that the electric and magnetic field strengths are related by the equation B = E/c. Therefore, you only need one of these values to calculate the intensity of an electromagnetic wave.
Example Calculation
Let’s assume we have an electromagnetic wave with an electric field strength (E) of 50 V/m. We can calculate the intensity (I) using the formula:
I = 1/2 μ0 c E2
Plugging in the values, we get:
I = 1/2 × (4π × 10-7 N/A2) × (3 × 108 m/s) × (50 V/m)2
Upon simplification, the intensity (I) is found to be approximately 1.33 × 10-3 W/m2.
In conclusion